
| Designated Hitter | January 18, 2010 |
The game of baseball as played today at the amateur level is very different from the game I played growing up in Rumford, Maine in the early 1960s. In my youth, wood bats ruled. Nowadays, almost no one outside the professional level uses wood bats, which have largely been replaced by hollow metal (usually aluminum) or composite bats. The original reason for switching to aluminum bats was purely economic, since aluminum bats don’t break. However, in the nearly 40 years since they were first introduced, they have evolved into superb hitting instruments that, left unregulated, can significantly outperform wood bats. Indeed, they have the potential of upsetting the delicate balance between pitcher and batter that is at the heart of the game itself. This state of affairs has led various governing agencies (NCAA, Amateur Softball Association, etc.) to impose regulations that limit the performance of nonwood bats. The primary focus of this article is on the techniques used to measure and compare the performance of bats.
Any discussion of bat performance needs to begin with a working definition of the word “performance.” Or, said a bit differently, what is meant by the statement, “bat A outperforms bat B”? Among people who have thought about this question, a consensus has emerged that a good working definition of performance is batted ball speed (or simply BBS). Generally speaking, if you want to improve your chances of getting a hit, then you want to maximize BBS, regardless of whether you are swinging for the fences or just trying to hit a well-placed line drive through a hole in the infield. The faster the ball comes off the bat, the better are your chances of reaching base safely. So, we will say that bat A outperforms bat B if the batter can achieve higher BBS with bat A than with bat B.
Which then brings up the next question: What does BBS depend on? I answer that by writing down the only formula you will find in this article:
This “master formula” is remarkably simple in that it relates the BBS to the pitch speed, the bat speed, and a quantity q that I will discuss shortly. It agrees with some of our intuitions about batting. For example, we know that BBS will depend on the pitch speed, remembering the old adage that `'the faster it comes in, the faster it goes out.'' We also know that a harder swing—i.e., a larger bat speed--will result in a larger BBS. All the other possible things besides pitch and bat speed that BBS might depend on are lumped together in q, which I will call the “collision efficiency.” As the name suggests, q is a measure of how efficient the bat is at taking the incoming pitch, turning it around, and sending it along its merry way. It is an important property of a bat. All other things equal, when q is large, BBS will be large. And vice versa. For a typical 34-inch, 31-oz wood bat impacted at the “sweet spot” (about 6 inches from the tip), q is approximately 0.2, so that the master formula can be written BBS = 0.2*(pitch speed) + 1.2*(bat speed). This simple but elegant result tells us something that anyone who has played the game knows very well, at least qualitatively. Namely, bat speed is much more important than pitch speed in determining BBS. Indeed, the formula tells us that bat speed is six times more important than pitch speed, a fact that agrees with our observations from the game. For example, we know that a batter can hit a fungo a long way (with the pitch speed essentially zero) but cannot bunt the ball very far (with the bat speed zero). Plugging in some numbers, for a pitch speed of 85 mph (typical of a good MLB fastball as it crosses home plate) and a bat speed of 70 mph, we get BBS=101 mph, which is enough to carry the ball close to 400 ft if hit at the optimum launch angle. Each 1 mph additional pitch speed will lead to about another 1 ft, whereas an extra 1 mph of bat speed will result in another 6 ft. On the other hand, if the bat were a “hotter bat” with q=0.22, that would add 3 mph to BBS, adding a whopping 18 ft to a long fly ball.
The master formula tells us that the quantities that determine bat performance are the collision efficiency and the bat speed, leading us to ask our next question. What specific properties of a bat determine its bat speed and collision efficiency? There are two such properties: the ball-bat coefficient of restitution (BBCOR) and the moment of inertia (MOI). In the following paragraphs, I’ll explain what these properties are and how they contribute to bat performance. The interplay among the various quantities is shown schematically in the picture below.
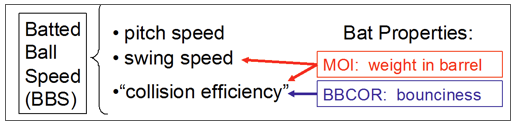
 Let’s start with the BBCOR, which is a measure of the “bounciness” of the ball-bat collision. First a brief digression. During a high-speed ball-bat collision, the ball compresses by about 1/2 of its natural diameter and sort of wraps itself around the bat, as shown in the accompanying photo. It then expands back out again, pushing against the bat. During this process, much of the initial energy of the ball is converted to heat due to the friction from the rubbing of threads of yarn against each other. Try dropping a baseball onto a hard rigid surface, such as a solid wood floor. The ball bounces to only a small fraction of its initial height, reflecting the loss of energy in the collision with the floor. A wood bat with its solid barrel behaves more or less like a rigid surface. But a hollow aluminum bat is different since it has a thin flexible wall that can “give” when the ball hits it. Some of the ball’s initial energy that would otherwise have gone into compressing the ball instead goes into compressing the wall of the bat. The more flexible the wall, the less the ball compresses and therefore the less energy lost in the collision. This process is commonly called the “trampoline effect,” and the BBCOR is simply a quantitative measure of that effect. A wood bat has essentially no trampoline effect and has a BBCOR ≈ 0.50. Hollow bats can have a substantially larger BBCOR, leading to a larger q and a correspondingly larger BBS. For example, a bat with BBCOR = 0.55 will have about a 5 mph larger BBS. Indeed, the technology of making a modern high-performing bat is aimed primarily at improving the trampoline effect—i.e., increasing the BBCOR and consequently the BBS. For aluminum this is achieved by developing new high-strength alloys that can be made thinner (to increase the trampoline effect) without denting. The past decade has seen the development of new composite materials that increase the barrel flexibility beyond that achievable with aluminum, giving rise to a new generation of high-performing bats.
Let’s start with the BBCOR, which is a measure of the “bounciness” of the ball-bat collision. First a brief digression. During a high-speed ball-bat collision, the ball compresses by about 1/2 of its natural diameter and sort of wraps itself around the bat, as shown in the accompanying photo. It then expands back out again, pushing against the bat. During this process, much of the initial energy of the ball is converted to heat due to the friction from the rubbing of threads of yarn against each other. Try dropping a baseball onto a hard rigid surface, such as a solid wood floor. The ball bounces to only a small fraction of its initial height, reflecting the loss of energy in the collision with the floor. A wood bat with its solid barrel behaves more or less like a rigid surface. But a hollow aluminum bat is different since it has a thin flexible wall that can “give” when the ball hits it. Some of the ball’s initial energy that would otherwise have gone into compressing the ball instead goes into compressing the wall of the bat. The more flexible the wall, the less the ball compresses and therefore the less energy lost in the collision. This process is commonly called the “trampoline effect,” and the BBCOR is simply a quantitative measure of that effect. A wood bat has essentially no trampoline effect and has a BBCOR ≈ 0.50. Hollow bats can have a substantially larger BBCOR, leading to a larger q and a correspondingly larger BBS. For example, a bat with BBCOR = 0.55 will have about a 5 mph larger BBS. Indeed, the technology of making a modern high-performing bat is aimed primarily at improving the trampoline effect—i.e., increasing the BBCOR and consequently the BBS. For aluminum this is achieved by developing new high-strength alloys that can be made thinner (to increase the trampoline effect) without denting. The past decade has seen the development of new composite materials that increase the barrel flexibility beyond that achievable with aluminum, giving rise to a new generation of high-performing bats.
We now turn to the MOI, which depends on both the weight of the bat and the distribution of the weight along its length. For a given weight, the MOI is largest when a larger fraction of the weight is concentrated in the business end of the bat (i.e., the barrel). The MOI affects bat performance in two ways in that both q and the bat speed depend on it. A larger MOI means a larger q (and vice versa), in complete agreement with our intuition. A heavier bat will be more efficient than a light bat in transferring energy to the ball. But, contrary to popular belief, it is not the total weight of the bat that matters but rather the weight in the barrel, where the collision with the ball occurs. That’s why it is the MOI that matters and not just the weight. But a larger MOI also means that the bat won’t be swung as fast, which again agrees with our intuition. Once again, research has shown that it is the MOI of the bat and not just the weight that affects swing speed.
The fact that the MOI affects bat performance in two opposite ways raises an interesting question. If I have two bats with the same BBCOR but with different MOI, which one will have the larger BBS? For example, if I “cork” a wood bat, which reduces its MOI, will the resulting increase in swing speed compensate for the reduction in collision efficiency? Current research suggests that the answer is “no” and that corking a bat does not lead to a larger BBS. For a detailed account, see this article. By the way, corking a wood bat does have some important advantages, even though higher BBS is not one of them. By reducing the MOI, the batter will have a “quicker” and more easily maneuverable bat, allowing him to wait a bit longer on the pitch and to make adjustments once the swing has begun. So, although corking a bat may not lead to higher BBS, it certainly may lead to better contact more often.
For bats of a given length and weight, the MOI will generally be smaller for an aluminum bat than for a wood bat. After all, a wood bat is a solid object, so a larger fraction of its weight is concentrated in the barrel than for a hollow nonwood bat. Here is another simple experiment you can do. Take two bats of the same length and weight (e.g., 34”, 31 oz), one wood and one aluminum, and find the point on the bat where you can balance it on the tip of your finger. You will find that the balance point is farther from the handle for the wood bat than for the aluminum bat, showing that a larger concentration of the weight is in the barrel for the wood bat. However, keeping in mind the corked bat discussion, the lower MOI for an aluminum bat results in no net advantage or disadvantage for BBS. The real advantage in BBS of aluminum over wood is in the BBCOR (i.e., the trampoline effect).
Let’s talk briefly about how bat performance is measured in the laboratory. Details can be found at this web site. Briefly, the basic idea is to fire a baseball from a high-speed air cannon at speeds up to about 140 mph onto the barrel of a stationary bat that is held horizontally and supported at the handle. Both the incoming and rebounding ball pass through a series of light screens, which are used to measure accurately its speed. The collision efficiency q is the ratio of rebounding to incoming speed. The MOI is measured by suspending the bat vertically and allowing it to swing freely like a pendulum while supported at the handle. The MOI is related to the period of the pendulum. Once q and the MOI are known, these can be plugged into a well-established formula to determine the BBCOR. To calculate BBS, the master formula is used along with a prescription for specifying the pitch and bat speeds, the latter of which will depend inversely on the MOI.
Various organizations use this information in different ways to regulate the performance of bats. The Amateur Softball Association regulates BBS, using laboratory measurements of q and MOI along with the prescriptions noted above to calculate BBS using the master formula. For the past decade, the NCAA has regulated baseball bats by requiring that q is below some maximum value and the MOI is above some minimum value, the latter limiting the swing speed. Together the upper limit on q and lower limit on the MOI effectively limit the maximum BBS. The maximum q is set to be the same for nonwood as for wood. The lower limit on MOI is such that the best-performing nonwood bat outperforms wood by about 5 mph. You may have seen the words “BESR Certified” stamped on NCAA bats. The BESR is shorthand for the Ball Exit Speed Ratio; numerically, BESR = q + 1/2. Starting in 2011, the NCAA will instead regulate the BBCOR, taking advantage of the fact that for bats of a given BBCOR, the BBS does not depend strongly on MOI. Moreover, the NCAA has set the maximum BBCOR to be right at the wood level, so it is expected that nonwood bats used in NCAA will perform nearly identically to wood starting next year.
Alan Nathan has been a Professor of Physics at the University of Illinois since 1977. His research specialty is experimental nuclear/particle physics, with over 80 publications in scientific journals to his credit. He is a Fellow of the American Physical Society. For the last decade he has added the physics of baseball to his research portfolio and has written numerous papers on the subject for scientific journals, primarily on the physics of the ball-bat collision and the aerodynamics of baseball in flight. In addition, he has given many talks on the subject to both scientific and popular audiences and maintains a "physics of baseball" web site that is visited frequently. He is Chair of SABR's Baseball & Science Committee and a member of the scientific panel that advises the NCAA on issues related to bat performance.
Comments
I've heard stories that MLB hitters in the '50s used heavier wood bats than the current pros, and that part of the better hitting environment of the recent years can be explained by that. Is that true?
You seem to say in the article that the weight of the bat doesn't seem to matter much.
Posted by: ekogan at January 18, 2010 4:35 AM
Great article Alan. You mentioned that MOI is one of the factors that determines bat speed. Are there studies that have been done that discuss the relative importance of other factors? Are there other bat factors, such as total weight, circumference of the barrel, flexibility of the shaft? I would guess that physical properties of the batter would also play a large part as would techniques of the swing itself. But what are the relative importance of the various physical characteristics of the batter? Do height, weight, arm length, strength of different muscle groups (wrist, arms, legs, trunk) all play a part, and if so which are the most important? Similarly, what aspects of batting technique have been fouond to be most important to bat speed? Finally, has anyone studied the range of bat speeds for major league players? Or minor league or college players for that matter? I am wondering whether the ramge of player's maximum bat speeds is relatively wide or narrow.
Posted by: Peter Jensen at January 18, 2010 6:58 AM
How realistic is shift from 0.20 to 0.22? Would, say, a change from an ash bat to a maple bat plausibly cause that shift? What about different length/weight combinations?
Posted by: Mitch at January 18, 2010 7:49 AM
I responded to Mitch over at The Book, where he posted the identical question. Here is what I wrote there:
A shift from 0.20 to 0.22 is not only realistic, it is a modest shift. There are two ways to cause that shift. One is to make the BBCOR larger, which would require using a nonwood bat. Careful studies have shown that there is no difference in BBCOR between ash and maple. The second way is to increase the MOI. The latter would be partially compensated by a decrease in swing speed. A maple bat of the same dimensions as an ash bat (same length and radius profile) would have a larger MOI since maple is a bit more dense than ash. So, switching from ash to maple would be sort of like “anti-corking”, the opposite of corking. Since corking results in a slightly lower BBS, then anti-corking results in a slightly higher BBS, at the cost of less quickness.
Posted by: Alan Nathan at January 18, 2010 9:41 AM
Response to ekogan:
For bats of a given BBCOR, weight (i.e. MOI) matters only a little in terms of BBS. As a general rule, NCAA players tend to use bats with a lower-than-optimum MOI, sacrificing maximum BBS for better bat control. There is a tendency these days for MLB players to do the same.
Response to Peter:
I was one of the researchers on a study of swing speed for elite slow-pitch softball players. Download the report http://webusers.npl.illinois.edu/~a-nathan/pob/SwingSpeed.pdf">here
We tested two different categories of bats. First, bats of the same weight but different MOI; second, bats of the same MOI but different weight. We found swing speeds varied inversely with MOI for the first group and were independent of weight for the second group. No other characteristics of the bat were varied other than weight and MOI.
One surprising result from the study was that these elite softball players have swing speeds that approach 100 mph at the actual ball-bat contact point. While there have not been as careful studies done with MLB players, I would bet there is not a single MLB player with a swing speed that high in game conditions. Of course, with slow-pitch softball, bat quickness is not such a big issue and the batter can basically swing as hard as he can with no real penalty. If he did that in a MLB game, he would probably swing and miss most of the time. But every once in a while, he would get lucky and hit the ball a very long distance.
Posted by: Alan Nathan at January 18, 2010 9:57 AM
Thanks, Alan. The differences between wood and aluminum are pretty obvious to naked eye, but I was curious if bat technology on wood-only models could cause dramatic shifts.
Posted by: Mitch at January 18, 2010 11:41 AM
Thanks Alan nice piece on explaining the differences in bats and what is going on in the NCAA. However I have to take issue with "Moreover, the NCAA has set the maximum BBCOR to be right at the wood level, so it is expected that non-wood bats used in NCAA will perform nearly identically to wood starting next year."
First non-wood bats will never behave like wood bats because they have a larger sweet spot and therefore perform better on a miss hit balls. Second they do not break so they prevent pitchers from pitching inside more in the college game, which dramatically changes the college game. Finally, why doesn't the NCAA stop all this nonsense and just go back to wood? It can no longer be considered economic since composite and aluminum bats cost nearly $400. A player (or college) can afford to purchase several bats over the season at or under the $400. There are also hybrid wood bats that perform like wood that are not as breakable. I for one look forward to the day the NCAA wakes up and puts the college game back where it belongs and mandates wood or wood equivalent bats.
Posted by: joebush at January 22, 2010 11:31 AM
Re joebush: In the context of my definition of "performance", my statement is completely accurate. With the new BBCOR rule, the maximum batted ball speed will be the same for wood and nonwood. Certainly I agree that even with the same BBCOR, wood and aluminum bats are different hitting instruments. The "larger sweet spot" thing is an interesting discussion all by itself, but let me save that for another time.
Posted by: Alan Nathan at January 22, 2010 11:57 AM